Many mathematical concepts from vector analyzes that energy is scalar or vector must be used to better grasp the theory of propulsion. Most people are introduced to secondary and secondary education vectors, but the mathematically challenge is for elementary and middle school students:
Is energy scalar or vector? In this article we are going to examine this question. A vector analysis has many complex parts and we won’t go there. We will only concentrate on the essential issues. Vectors permit one to view complex , multi-dimensional issues as a single-dimensional problem category. We are mainly concerned with definitions. The words are a bit strange, but as you’ll see, the ideas are very strong. You can read this article on vector analysis if you want to find out even more about vectors.
People also invented math and science to represent and appreciate the world around us. We live in a 4-D (at least) universe controlled by time and three dimensions of space; up , down, left, right, back and forth. We note that in our world, certain quantities and processes depend on the direction in which they take place, and some quantities do not depend on the direction. The volume of the object , for example, the three-dimensional space occupied by an object does not depend on direction. We also have a 5 cubic foot iron block and we have a 5 cubic foot iron block and we push it up and down and then left and right.
The location, on the other hand, depends on the direction of an object. The effect is a very different place if we move the 5 cubic footblock 5 miles North, than if we move it 5 miles East. A number based on the direction of a vector quantity is named by mathematicians and science experts. Scalar quantity is called a quantity that does not depend on direction.
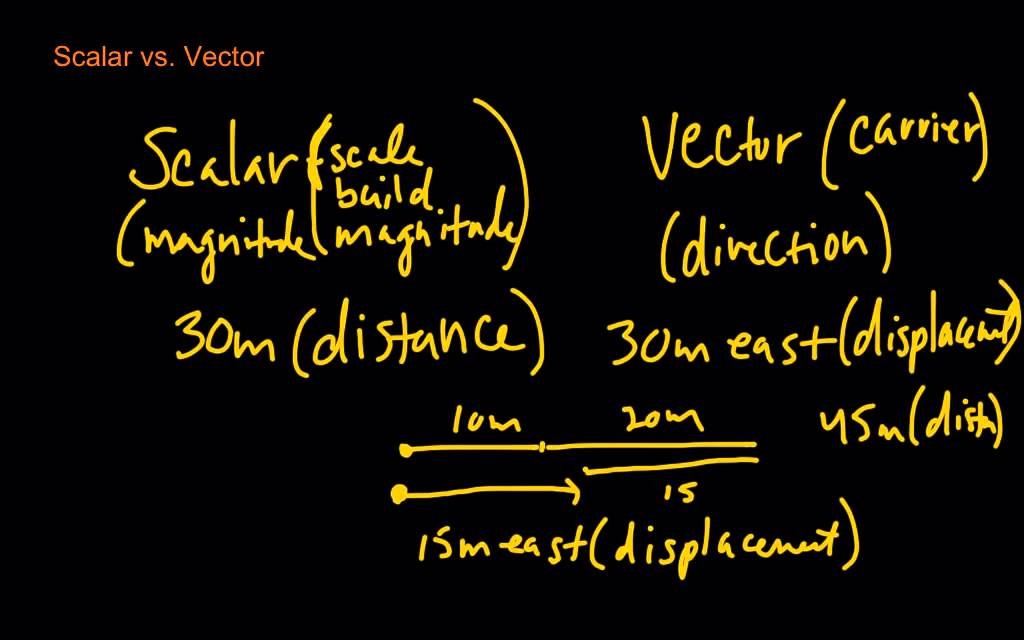
The quantity of the vector has two characteristics, one size and one direction. Scalar quantities have a scale only. You should compare the magnitude and the direction in comparing two vector quantities of the same type. Even the magnitude must be applied to scalars. You have to remember the magnitude and the direction when performing all mathematical operations on a vector number (such as adding , subtracting, multiplying …). This makes it a little more complicated than scalars to handle vector quantities.
On the slides we listed some of the physical volumes discussed in the Aeronautics Guide and grouped into vector or scalar numbers. Of particular importance are the forces, weight, thrust and aerodynamic forces that are active on a flying aircraft, all in a vector quantity. The resulting motion, speed and acceleration of the aircraft are both vector numbers. Those quantities can be calculated by the vector laws of Newton. The scalar quantities provide the majority of thermodynamic states that are related to the propulsion system, such as the propellant density, pressure and temperature. Scalar quantities are also the energy, function and entropy associated with the motors.
If we want to know answer that is energy scalar or vector, Scale and direction of the vectors, magnitude of just the scalars. It may lead to some misunderstanding that the magnitude is present for both scalars and vectors. There are certain quantities, such as speed, that have very special scientist definitions. By definition, the velocity of a vector is the scalar magnitude. The speed of a car entering the road is 50 mph. It has a speed of 50 mph in the direction north-east. When the words are used interchangeably, it can become very confusing! Mass and weight are another example. Weight is a vector force with magnitude and direction. It’s a scalar Mass. Weight and mass are interrelated, but not the same amount.
While the laws of Newton describe a solid ‘s subsequent motion, special equations are present that characterize fluids, gases and liquids. The mass, dynamics and energy of the system must be conserved for every physical system. Electricity and mass are scalar, and vector quantities are momentum. This leads to a combination of equations, known as Navier Stokes, which describe what liquids do when exposed to external forces. These equations are fluid and very difficult to resolve and to understand Newton ‘s movements. For certain fluid problems, a simplified version of the Euler equations can be resolved.